BELIEVE   ME   NOT!    - -     A   SKEPTICs   GUIDE  

Black Holes

As long as we're being relativistic, why not go all the way? Suppose a very lightweight particle is in orbit around a very heavy mass m, attracted only by gravity. A simple application of Newton's Second Law yields the ORBITAL VELOCITY

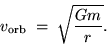$$v_{\rm orb} \; = \; \sqrt{G m \over r} .$$ (24.18)

Taking this at face value, what happens when $v_{\rm orb} \to c$? For a given m, there is a radius called the SCHWARZSCHILD RADIUS

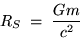$$R_S \; = \; {G m \over c^2 }$$ (24.19)

for which anything close to the mass cannot maintain its orbit without exceeding the speed of light. Since this is impossible [I am being really sloppy and glib now, but the conclusion is qualitatively correct] once anything gets inside that radius it falls in the rest of the way and never comes out. Even light. Hence the term, `` BLACK HOLE''. Any mass m has its R_s; but usually the density of a given lump of matter is not high enough to place sufficient m inside a given r to cause a black hole to form.