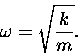BELIEVE   ME   NOT!    - -     A   SKEPTICs   GUIDE  

Damped Harmonic Motion

Let's take stock. In the previous chapter we found that

$$x(t) \; = \; \hbox{\rm [constant]} \; - \; {v_0 \over \kappa} \, e^{- \kappa \, t}$$

satisfies the basic differential equation

$$\ddot{x} = - \kappa \dot{x} \qquad \hbox{\rm or} \qquad a = - \kappa v$$

defining damped motion (e.g. motion under the influence of a frictional force proportional to the velocity). We now have a solution to the equation of motion defining SHM,

$$\ddot{x} = - \omega^2 \, x \quad \Rightarrow \quad x(t) = x_0 \, e^{i \, \omega \, t} ,$$

where

$$\omega = \sqrt{k \over m} .$$

Can we put these together to ``solve'' the more general (and realistic) problem of damped harmonic motion? The differential equation would then read

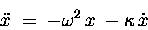$$\ddot{x} \; = \; - \omega^2 \, x \; -\kappa \, \dot{x}$$ (13.20)

which is beginning to look a little hard. Still, we can sort it out: the first term on the RHS says that there is a linear restoring force and an inertial factor. The second term says that there is a damping force proportional to the velocity. So the differential equation itself is not all that fearsome. How can we ``solve'' it?

As always, by trial and error. Since we have found the exponential function to be so useful, let's try one here: Suppose that

$$x(t) = x_0 \, e^{K \, t}$$ (13.21)

where  x₀  and  K  are unspecified constants. Now plug this into the differential equation and see what we get:

$$\dot{x} \; = \; K \, x_0 \, e^{K \, t} \; = \; K \, x$$

and

$$\ddot{x} \; = \; K^2 \, x_0 \, e^{K \, t} \; = \; K^2 \, x$$

The whole thing then reads

$$K^2 \, x \; = \; - \omega^2 \, x \; - \kappa \, K \, x$$

which can be true ``for all  x'' only if

$$K^2 = - \omega^2 \, - \kappa \, K \qquad \hbox{\rm or} \qquad K^2 + \kappa K + \omega^2 = 0$$

which is in the standard form of a general quadratic equation for  K,  to which there are two solutions:

$$K = { - \kappa \pm \sqrt{ \kappa^2 - 4 \omega^2 } \over 2 }$$ (13.22)

Either of the two solutions given by substituting Eq. (22) into Eq. (21) will satisfy Eq. (20) describing damped SHM. In fact, generally any linear combination of the two solutions will also be a solution. This can get complicated, but we have found the answer to a rather broad question.