
We will restrict our attention in the following to the long-time behaviour of AC circuits; in particular, we will examine the response of circuits at the same frequency as the driving source, neglecting any transient responses.
The voltage drop across a resistor (R), capacitor (C) and inductor (L) are given as:

We can represent the current by a complex exponential:
![]()
If we now evaluate ![]() ,
, ![]() and
and ![]() :
:
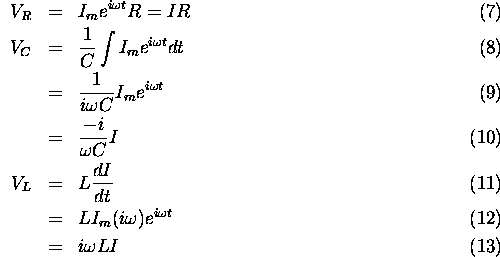
We can define the complex impedance X:

so that the voltage drop across any element is given simply as
![]()
Consider the circuit in Figure 9.6 consisting of a resistance R, a capacitance C and an inductance L, connected in series and driven by a time-varying voltage source.
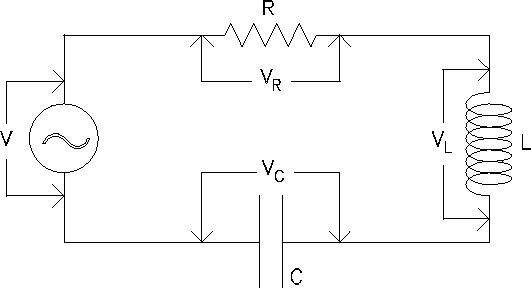
Figure 9.6: A driven series RLC circuit.
The differential equation for the current flow is obtained by equating the voltage of the source to the total potential drop around the circuit.
![]()
We write the driving sinusoidal voltage as ![]() , then
take the current to have the same frequency, but a relative phase
, then
take the current to have the same frequency, but a relative phase ![]() :
:
![]()
To determine the response of the circuit,
we need to determine ![]() .
.
Since the left hand side of the equation for ![]() is purely real,
the right hand side must also be real; in other words,
the imaginary part must equal zero.
Making use of the identity
is purely real,
the right hand side must also be real; in other words,
the imaginary part must equal zero.
Making use of the identity
![]() , we obtain:
, we obtain:
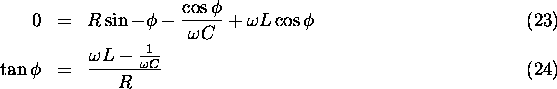
From our result for ![]() , we can construct a triangle
and use the Pythagorean theorem to obtain
, we can construct a triangle
and use the Pythagorean theorem to obtain
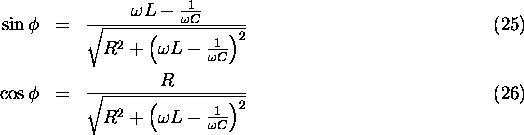
Substituting into the real part of Eq. (22) we obtain:
